Effect of time-reversal on the first principle of Granger causality
Investigators: Martina Chvosteková, Anna Krakovská, Jozef Jakubík
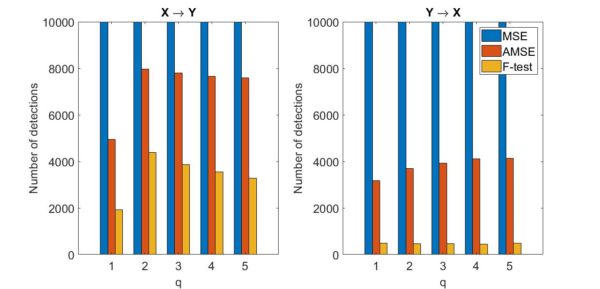
Identifying the causal link between processes in a complex system is one of the fundamental challenges in the natural and social sciences. The first principle of Granger causality as the basic quantitative analysis of causality is the chronological order of cause and effect. The effect of the time reversal of the processes generated by the linear autoregressive model on the first principle was analyzed in [1]. We have shown that the process that was the cause for the second process in the original time direction became a consequence after the time reversal of both processes, and at the same time remained the cause of the second process depending on the length of observations and predictability of processes. The possibility of using this result to investigate the interconnection in the system is still limited due to the lack of a degree of prediction accuracy suitable for causal analysis, which was pointed out in [2,3]. We have shown that the commonly used mean square error is not an appropriate measure of accuracy and we have analyzed the proposed adjusted mean square error. In [2], the negative effect of data smoothing on causal linking by measuring prediction accuracy was explained.
Fig.: Causal link analysis using Mean Squared Error, Adjusted Mean Squared Error, and F-test at a significance level of 0.05 for 10000 simulations of unidirectionally linked processes X and Y, where X is causal for Y. The number is correct. detected X-> Y links (on the left) and the number of incorrectly detected Y-> X links (on the right).
Related projects: VEGA-2/0081/19, VEGA-2/0096/21.
Publications:
- CHVOSTEKOVÁ, Martina – JAKUBÍK, Jozef – KRAKOVSKÁ, Anna. Granger causality on forward and reversed time series. In Entropy, 2021, vol. 23, no. 4, p. 409. ISSN 1099-4300. (2.524 – IF2020) Q2, ADCA
- CHVOSTEKOVÁ Martina – KRAKOVSKÁ Anna. Letter to the editor of Heliyon re: Grassmann, G. “New considerations on the validity of the Wiener-Granger causality test” [Heliyon 6 (2020)e05208]. In Heliyon, 2021, vol. 7, no. 9, e07948. ISSN 2405-8440. Q1, ADCB
- CHVOSTEKOVÁ, Martina. A measure of prediction precision for Granger causality analysis. In MEASUREMENT 2021 : Proceedings of the 13th International Conference on Measurement. – Bratislava : Institute of Measurement Science, SAS, 2021, p. 6-9. ISBN 978-80-972629-4-5. Q4, ADNB
 Contacts
Contacts Intranet
Intranet SK
SK